Here you will find all the formulas and numerous examples for calculating the area of triangles. The areas of both general and special triangles, such as right-angled or equilateral triangles, are considered. With the calculator for calculating the area of triangles, you can simply enter the given dimensions of a triangle and thus have the area of the triangle calculated. In addition to the display of the area calculated in this way, you will also receive detailed derivations of the area calculation in the result of the calculator.
On the page of our Triangle Calculator you will find numerous examples for the calculation of all characteristics of triangles. Or visit our guides on the topics 'Right-angled triangles' and 'Equilateral triangles'.
Contents on the topic 'Area of a Triangle'
Contents
- Definition of a Triangle
- Label of a Triangle
- Area of Triangles
- 1. Given a known side and an associated height h
- 2. Given three known sides
- 3. Given equilateral triangles with a known side
- 4. With two known sides and an angle enclosed
- 5. Given right triangles with known catheti
- 6. With one known side and two known angles
- 7. With two known sides and an angle opposite the longer side
Definition of a Triangle
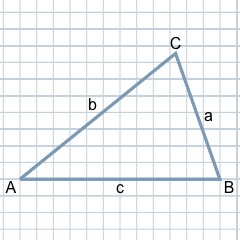
Before we go into the calculation of the area of a triangle, here is a brief definition of a triangle and a description of the general terms of a triangle.
A triangle is defined by three points in the plane that do not lie on a straight line. These three points are the corners of the triangle. Each connecting line between two corners is a side of the triangle. In the plane, the triangle thus delimits a surface.
Important properties of a triangle are its area, the length of its three sides, the perimeter of the triangle, the angles of the sides to each other and the heights of each side to the opposite corner.
You can easily calculate the area of a triangle and its other properties online using the calculator for calculating the area of a triangle. In the following section, you will also find all the options for calculating the area including the calculation formulas with examples.
How is a triangle labelled?
The three corners of a triangle are usually labelled with the capital letters A, B and C. This labelling is done in an anti-clockwise direction and usually begins with A at the bottom-left corner.
The three sides of a triangle are designated by the lower case letters a, b and c. Here, the side opposite corner A is labelled a. Accordingly, side b is opposite corner B and side c is opposite corner C.
The three inner angles of a triangle are named using the Greek letters α (alpha), β (beta) and γ (gamma). They are located at the corresponding corners: α belongs to corner A, β is at B and the angle γ is at C.
The height to one side corresponds to the perpendicular distance from the opposite corner point to this side or to its extension. Thus the height of a (ha) corresponds to the distance between the corner A and the opposite side a to which ha is perpendicular. Analogously, the height of b (hb) and the height of c (hc) are defined.
Introduction to the Area of a Triangles
To calculate the area of a triangle, the length of one side together with the corresponding height is sufficient. Using these two given values, the area of the triangle can be determined. We will deal with this case in the following section under 'Area of a triangle with a known side and a corresponding height h".
However, it often happens that the length of a side and the corresponding height are not known. Instead, other properties, such as the length of all three sides of the triangle, are given or other combinations of properties are given, which also enable the calculation of the area.
Following the first case mentioned, in which a base side and the corresponding height are known, we therefore go into six further combinations of given triangle properties under (2) to (7) on the basis of which the area of the triangle can be calculated. By the way, these combinations of given values, i.e., (2) to (7), are not only suitable for calculating the area of the triangle but are also suitable for calculating all parameters of the triangle using the combinations. Using these combinations, the total triangle can be calculated in addition to the area.
1. Area of a triangles with a known side and a corresponding height h
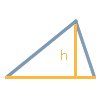This is the 'classic case' that is usually taught first in school lessons. Using the length of a base side g and the corresponding height h, the triangle area can be calculated with the help of a simple formula. However, these two pieces of information alone are not sufficient to determine a triangle unambiguously, i.e. to calculate the lengths of the other sides and thus construct the triangle. On the other hand, the following formula is best suited for calculating the total area.
Example
We will show you here how to calculate the area using an example where the side a of a triangle is given as a = 6 in and the height ha = 4 in.
Area formula for a given base side and height
A = ½ × g × hg
where A = Area, g = Length of the base side and h = Height to base side
Inserting the existing values
By inserting the values a = 6 for the base side and the height of a with ha = 4, we obtain
A = ½ × 6 × 4 = 12.
Solution
The area of the triangle is 12 in².
By the way, each of the three sides a, b and c with their corresponding heights h, can be used to calculate the area A.
The respective base side and the height must of course be given in the same unit of measurement. If, for example, the base side is given in mm and the height in inch, they must first be converted to one of the two units of measurement before the area of the triangle can be calculated. Of course, this also applies to all further area calculations that follow.
2. Area of a triangle with three known sides
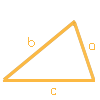Once all three sides of a triangle are given, all its properties can also be calculated. We would like to present Heron's formula for calculating the area of the triangle here.
Example
We will show you here how to calculate the area of the triangle with an example where the sides are given as a = 4 in, b = 6 in and c = 6 in.
Area formula (Heron's formula)
A = s(s − a)(s − b)(s − c)
where s = half the circumference, i.e.
s = (a + b + c) / 2
Inserting the existing values
Inserting the values a = 4, b = 6 and c = 6, we first obtain s with
s = (4 + 6 + 6) / 2 = 8
If one substitutes s = 8 into Heron's formula, one finally obtains
A = 8 × (8 − 4) × (8 − 6) × (8 − 6)) ≈ 11.31
Solution
The area of the triangle with three known sides is about 11.31 in².
3. Area of an equilateral triangle with a known side
If the length of one side of an equilateral triangle is known, we naturally know the length of all three sides, because the two remaining sides also have the same length. Thus we finally have a triangle with three known sides. We could now proceed as in 'area of triangles with three known sides' using Heron's formula to calculate the area of the triangle. However, this formula is simplified due to the fact that all three sides are of equal length.
Example
We will show you here how to calculate the area using an example where the equilateral triangle has a side length of a = 5 in.
Area formula for equilateral triangle
A = 3 / 4 × a²
Inserting the existing values
If you insert a = 3 into the formula, you get
A = 3 / 4 × 5² ≈ 10.83
Solution
The area of the equilateral triangle is about 10.83 in².
4. Area of a triangle with two known sides and an angle enclosed by them
With two known sides and the angle enclosed by them, all the properties and thus also the area of a triangle can be calculated.
For example, one could now first determine the height to one of the known sides (e.g., For known sides a and b with an included angle γ the height to b using hb = a × sin γ) and then, as described in 'Area for a triangle with a known side and associated height h', use the formula F = ½ × b × hb.
Here, however, we first want to calculate the missing third side using the cosine theorem and then, as described in 'Area of a trianglea with three known sides', use Heron's formula to calculate the area of the triangle with all three sides known.
Example
The area is calculated here using an example where the two sides a = 4 in and b = 6 in and the angle γ enclosed by a and b is 70 degrees.
Formula for calculating the missing side (cosine theorem)
The general cosine theorem for the missing side c is
c² = a² + b² − 2ab × cos γ°
and thus
c = a² + b² − 2ab × cos γ°
Inserting the existing values
If we insert the values for a, b and gamma, we obtain
c = 4² +6² − 4 × 6 × cos 70° ≈ 5.97
Interim solution
The missing side c of the triangle with two known sides and a known included angle is about 5.97 in.
Now that the lengths of all three sides are given as a = 4 in, b = 6 inand c = 5.97 in, "Heron's formula" can be applied to calculate the area. Thus
Area formula (Heron's formula)
A = s(s − a)(s − b)(s − c)
where s = half the circumference, i.e.
s = (a + b + c) / 2
Inserting the existing values
Inserting the values a = 4, b = 6 and c = 5.97, we first obtain s with
s = (4 + 6 + 5.97) / 2 ≈ 7.98
If we insert s = 7.98 into Heron's formula, we finally obtain
A = 7.98 × (7.98 − 4) × (7.98 − 6) × (7.98 − 5.97)) ≈ 11.28
Solution
The area of the triangle with two known sides and a known included angle is about 11.28 in².
5. Area of right-angled triangles with known cathets
If, in the case of a right triangle, the lengths of the two catheti lying at the right angle are known, then we have, in fact, a special case of the previous procedure in 'Area of triangles with two known sides and an angle enclosed by them', since the angle enclosed by the two catheti is known and is 90 degrees.
We could now continue with the calculation there under 4, i.e., calculate first the length of the unknown side using the cosine theorem and then calculate the area of the triangle using Heron's formula. However, a right-angled triangle with two given cathets allows a much simpler calculation of the area.
Example
In this example, to calculate the area for the right triangle, we know its two catheti a and b, where a = 4 in and b = 5 in.
Area formula for right triangle
In a right triangle, the two known catheti (here a and b) enclose the right angle. Therefore the following formula applies to the area
A = ½ × a × b
Due to the right angle between the two known sides, the formula is very similar to the area formula used here under 'Area of a triangle with a known side and an associated height h' (A = ½ × g × hg). This is because in a right triangle the altitude of a is exactly b, or conversely, the altitude of the cathetus b is exactly a.
The area formula for the right triangle can be illustrated by duplicating it and placing the two triangles together at their longest side, the hypotenuse, so that a rectangle is formed. This rectangle has the area a × b (cathetus a times cathetus b). Thus, before duplicating, the triangle has exactly half the area, i.e. ½ × a × b.
Inserting the existing values
By substituting a = 4 and b = 5 into the area formula for the right triangle, we get
A = ½ × 4 × 5 = 10
Solution
The area of the right triangle is 10 in².
6. Area of a triangle with one known side and two known angles
For the constellation in which any one side and any two angles are known for a general triangle, a little more preliminary work must be done to calculate the area: In the following example, we first calculate the missing angle using the sum of angles. With the three angles than known, the two remaining sides can be determined using the sine theorem. Once all three sides are known, we can proceed as in 'Area of a triangle with three known sides' and calculate the area of the triangle using Heron's formula.
Example
Here is an example of calculating the area of a general triangle using the given side a = 4 in and the two angles α = 40 degrees and β = 70 degrees.
Angle sum theorem to calculate the missing angle γ
According to the angle sum theorem, the sum of the three interior angles α, β and γ in a triangle is always 180 degrees.
α° + β° + γ° = 180°
When converted to γ° the formula becomes
γ° = 180° − α° − β°
Inserting the existing values
Substituting the values for α = 40 degrees and β = 70 degrees into the angle sum theorem, we get
γ° = 180° − 40° − 70° = 70°
Interim solution
The missing angle γ of the triangle with two known angles is 70°.
Now the remaining two sides b and c can be determined using the existing side and angle. The sine theorem is used for this purpose.
Sine theorem for calculating the missing side b
sin β / b = sin α / a
When converted to b, the formula is
b = a × sin β / sin α
Inserting the existing values
If one substitutes a, α and β into the transformed sine theorem, one finally obtains
b = 4 × sin 70° / sin 40° = 5.85
Analogously, the length of c can also be calculated using the corresponding sine theorem for c.
Intermediate solution
The missing sides b and c are b = 5.85 in and also c = 5.85 in.
Now that all three sides are known, we can than proceed as in 'Area of a triangles with three known sides' and calculate the triangle's area using Heron's formula.
Area formula (Heron's formula)
A = s(s − a)(s − b)(s − c)
where s = half the circumference, i.e.
s = (a + b + c) / 2
Inserting the existing values
Inserting the values a = 4, b = 5.85 and c = 5.85, we first obtain s with
s = (4 + 5.85 + 5.85) / 2 = 7.85
If one substitutes s = 7.85 into Heron's formula, one finally obtains
A = 7.85 × (7.85 − 4) × (7.85 − 5.85) × (7.85 − 5.85)) ≈ 10.99
Solution
The area of the triangle with one known side and two known angles is about 10.99 in².
7. Area of a triangle with two known sides and known angle opposite the longer side
For a general triangle, where two sides and the angle opposite the longer side are known, it is possible to calculate a unique triangle and hence the area of the triangle. To calculate the area, however, as with the constellation of given values under (6), some calculations must be carried out in advance in order to finally calculate the area of the triangle:
In the following example, the missing angles are calculated first. The angle opposite the shorter side can be determined with the help of the sine theorem. The third angle can then be determined using the sum of angles. Now the missing side can be calculated again using the sine theorem based on the values already calculated, before finally proceeding as in 'Area of a triangle with three known sides' using Heron's formula to calculate the area of the triangle.
Example
Here is an example of calculating the area of a general triangle using two given sides a = 4 in and b = 6 in and the angle β = 70 degrees opposite the longer side.
The angle α opposite the shorter side a can be determined with the help of the following sine theorem.
Sine theorem to calculate the missing angle α
sin α / a = sin β / b
When converted to α the formula is
α = arccos( a × sin β° / b )
Inserting the existing values
Inserting the values for a = 4 in, b = 6 in and β = 70 degrees, we get
α = arccos( 4 × sin 70° / 6 ) ≈ 38.79°
Intermediate solution
The first missing angle α with respect to the shorter side a is about 38.79°.
Since the second angle α is now known, the third missing angle γ can be calculated using the angle sum theorem.
Angle sum theorem to calculate the missing angle γ
According to the angle sum theorem, the sum of the three interior angles α, β and γ in a triangle is always 180 degrees.
α° + β° + γ = 180°
When converted to γ° the formula is
γ° = 180° − α° − β°
Inserting the existing values
Substituting the values for α = 38.79 degrees and β = 70 degrees into the angle sum theorem, we get
γ° = 180° − 38.79° − 70° = 71.21°
Interim solution
The missing angle γ of the triangle with two known angles is 71.21°.
Now the remaining side c can be determined using the existing sides and angles. The sine theorem is used for this.
Sine theorem for calculating the missing side c
sin γ / c = sin β / b
When converted c, the formula is
c = b × sin γ / sin β
Inserting the existing values
If one substitutes b, β and γ into the transformed sine theorem, one finally obtains
c = 6 × sin 71.21° / sin 70.00° = 6.04
Interim solution
The missing side c is c = 6.04.
Now that all three sides are known, we can then proceed as in 'Area of a triangle with three known sides' and calculate the area of the triangle using Heron's formula.
Area formula (Heron's formula)
A = s(s − a)(s − b)(s − c)
where s = half the circumference, i.e.
s = (a + b + c) / 2
Inserting the existing values
Inserting the values a = 4, b = 5.85 and c = 5.85, we first obtain s with
s = (4 + 6 + 6.04) / 2 = 8.02
If we insert s = 8.02 into Heron's formula, we finally obtain
A = 8.02 × (8.02 − 4) × (8.02 − 6) × (8.02 − 6.04)) ≈ 11.36
Solution
The area of the triangle with one known side and two known angles is about 11.36 in².
More online calculators
Circle Calculation, Cylinder Calculation, Convert area units, Calculation Random Numbers, Fraction Calculator, Time Unit Converter, Percent Calculator, Calculator, Convert Length Units, Convert Roman Numerals
Source information
As source for the information in the 'Triangle' category, we have used in particular:
Last update
This page of the 'Triangle' category was last edited or reviewed by Michael Mühl on February 20, 2023. It corresponds to the current status.
Changes in this category "Triangle"
- 12.11.2022: Publication of an article Calculation of equilateral triangles.
- 12.11.2022: Publication of an article about Area of a triangle and about Right-angled triangles.
- 12.11.2022: Publication of the topic Calculate Triangle together with the corresponding texts.
- Editorial revision of this page